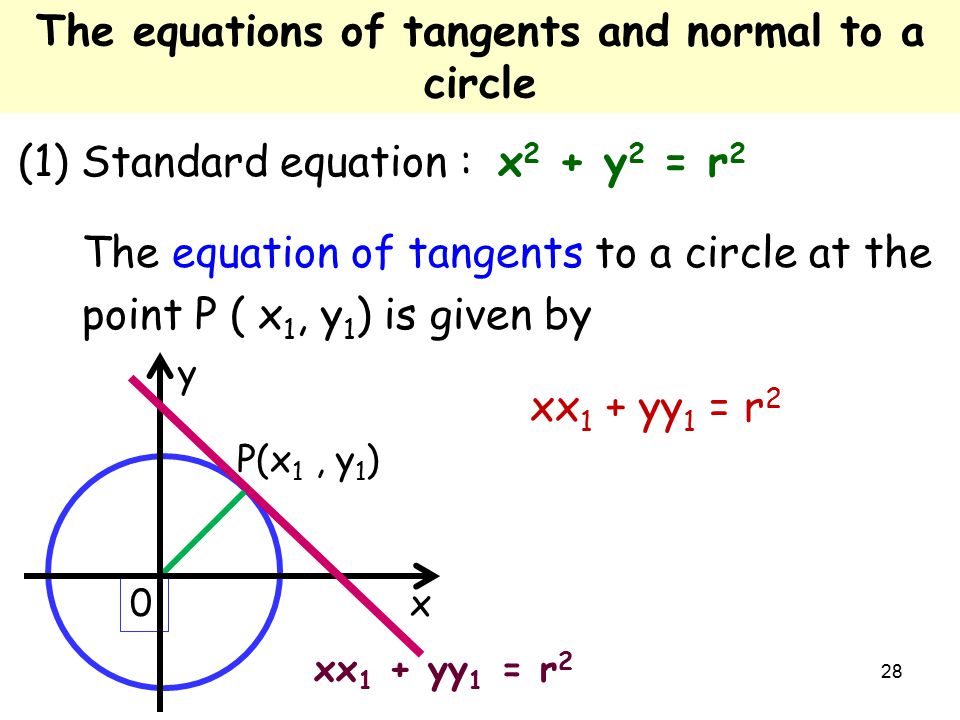
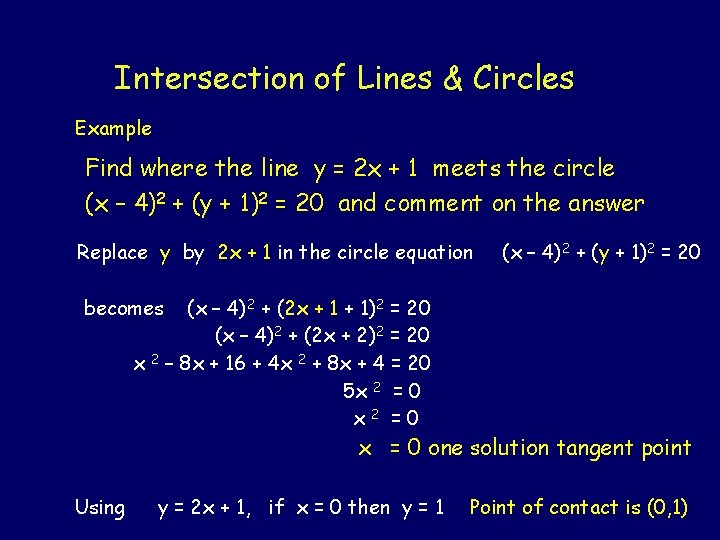
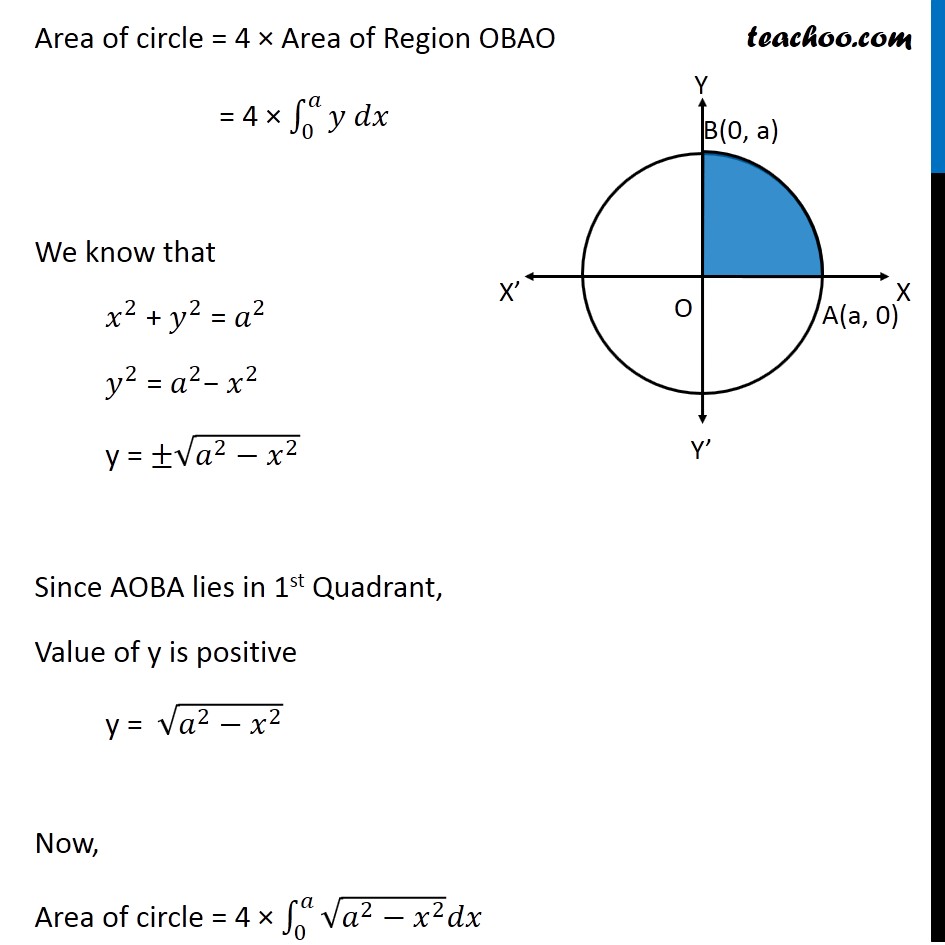
Equation of a normal to the circle x 2 y 2 = a 2 from a given point (x 1, y 1) In this case, the given normal will again pass through the point (x1, y1) and the center of the circle, except that the point (x1, y1) does not lie on the circle We'll use the the twoSuppose mathf(x,y) = x^2 y^2/math Let's look at the partial derivatives of this function math\displaystyle\frac{\partial f}{\partial x}= 2x/math mathAnswer Given equation of circle is x2 y2 = a2 ⇒ y2 = a2 −x2 ⇒ y = a2 −x2 ∴ Area of circle = 4×Area of first quadrant = 4∫ 0a
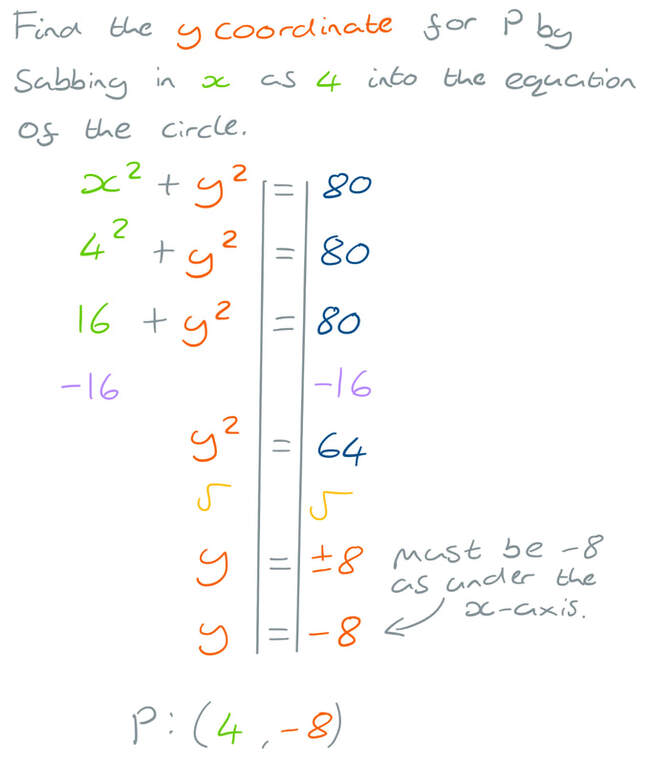
Q28 Answers Paper 3 June 18 Aqa Gcse Maths Higher Elevise
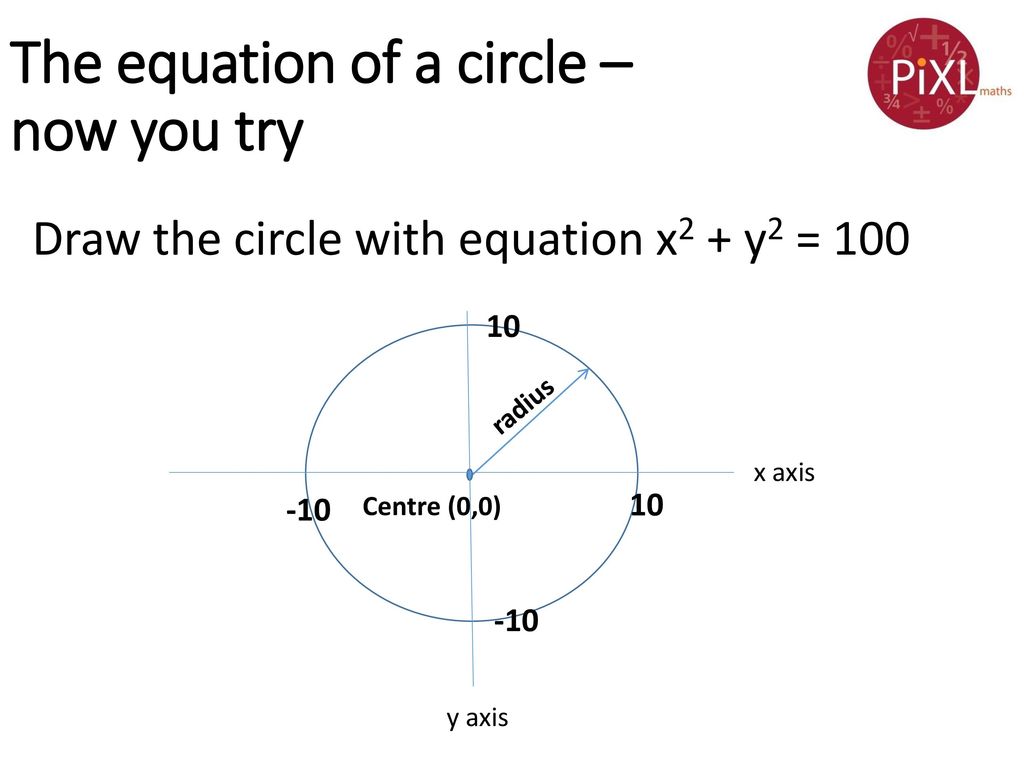
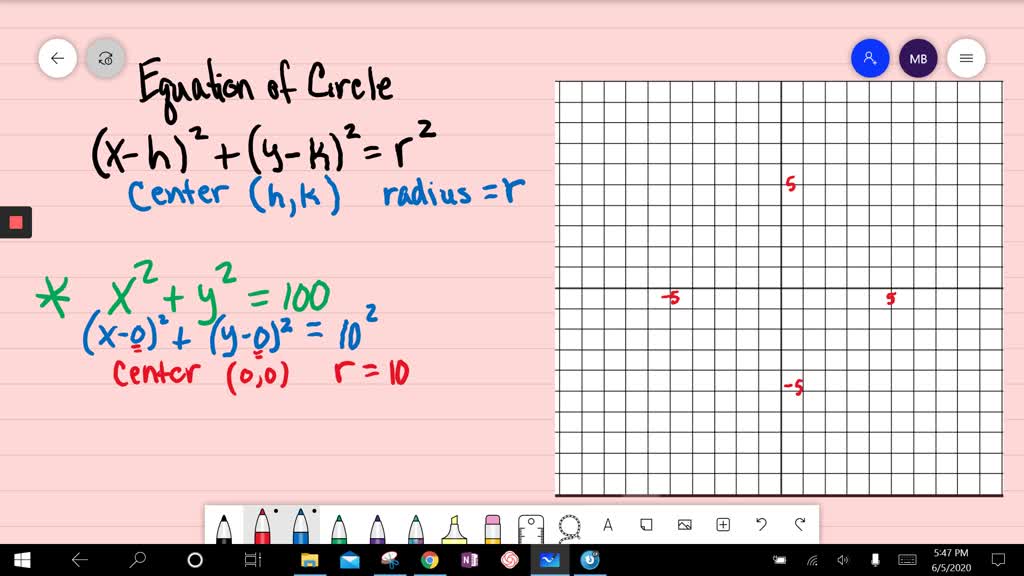
Circle c1 has equation x^2+y^2=100
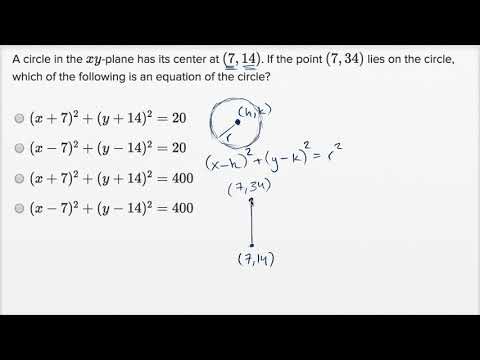
Circle c1 has equation x^2+y^2=100-Use the Distance Formula to find the equation of the circle ( x 2 − x 1) 2 ( y 2 − y 1) 2 = d Substitute ( x 1, y 1) = ( h, k), ( x 2, y 2) = ( x, y) and d = r ( x − h) 2 ( y − k) 2 = r Square each side ( x − h) 2 ( y − k) 2 = r 2 The equation of a circleAn ellipse is one of the shapes called conic sections, which is formed by the intersection of a plane with a right circular cone The general equation of an ellipse centered at (h, k) ( h, k) is (x − h)2 a2 (y − k)2 b2 = 1 ( x − h) 2 a 2 ( y − k) 2 b 2 = 1 when the major axis of the ellipse is horizontal



Recognise And Use X2 Y2 R2 Ppt Download
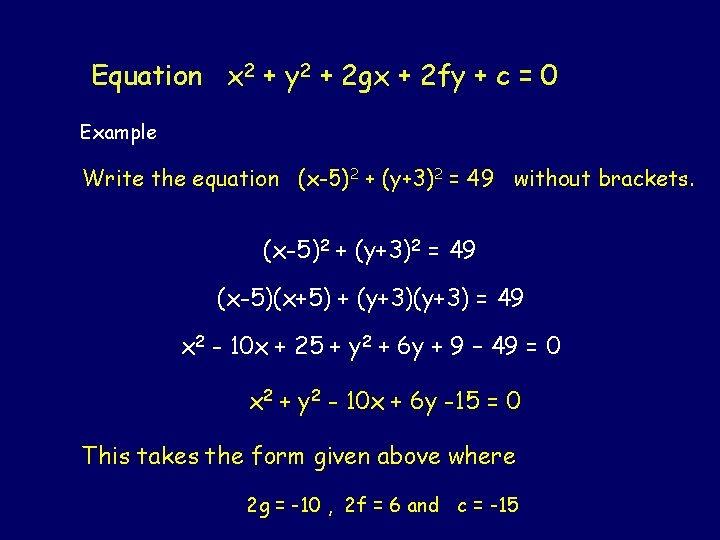
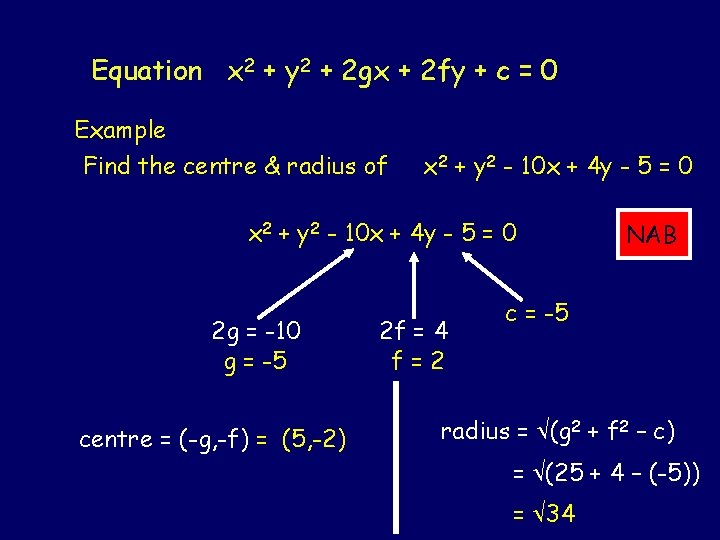
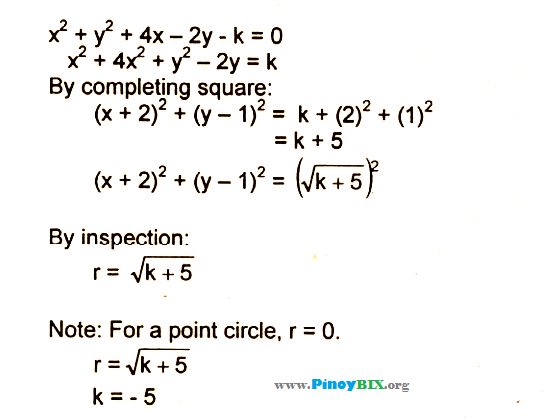
Answer is a way to express the definition of a circle on the coordinate plane The formula is ( x − h) 2 ( y − k) 2 = r 2 h and k are the x and y coordinates of the center of the circle ( x − 9) 2 ( y − 6) 2 = 100 is a circle centered at (9, 6) with a radius of 10If g 2 f 2 – c = 0, then the radius of the circle is 0 and the circle is known as point circleComparing the equation x^2y^212y45=0 with the general equation of the circle x^2y^22gx2fyc=0, we get 2g=0=> g = 0, 2f =12 => f = 12/2=6 and c= 45 Centre of the circle = (g,f) = (0,6) Radius of the circle =√ (g^2f^2c)
This lesson will cover a few examples to illustrate shortest distance between a circle and a point, a line or another circle Example 1 Find the shortest and the longest distance between the point (7, 7) and the circle x 2 y 2 – 6x – 8y 21 = 0 Solution We've established all the required formulas already in a previous lessonStill, have a look at what's going onWe know that the general equation for a circle is ( x h )^2 ( y k )^2 = r^2, where ( h, k ) is the center and r is the radius So add 21 to both sides to get the constant term to the righthand side of the equation x^2 y^2 4y = 21 Then complete the square for the y terms x^2 y^2SOLUTION The equation x^2y^2=169 defines a circle with its center at the origin and a radius of 13 The line y=x7 passes through the circle Determine the circle and line line intersect
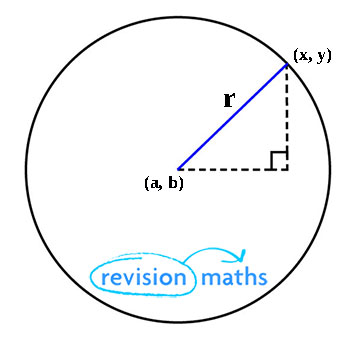
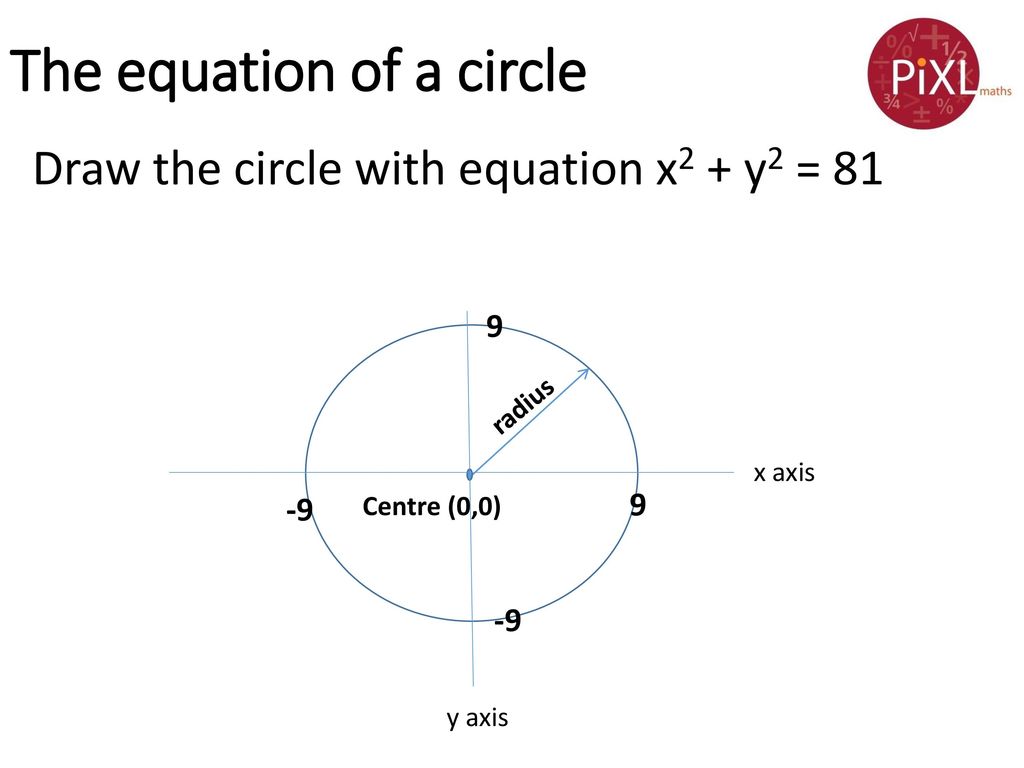
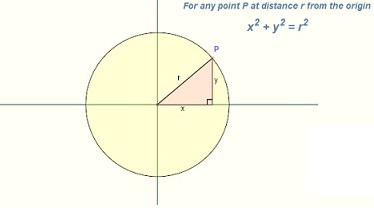
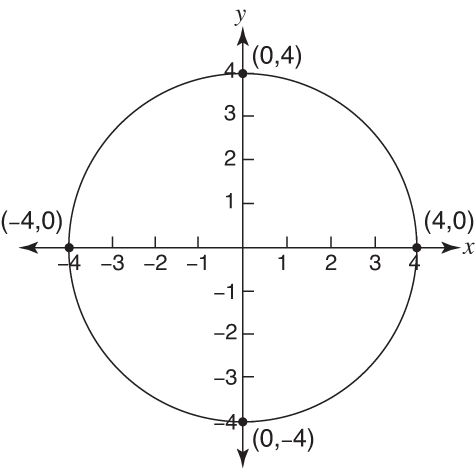
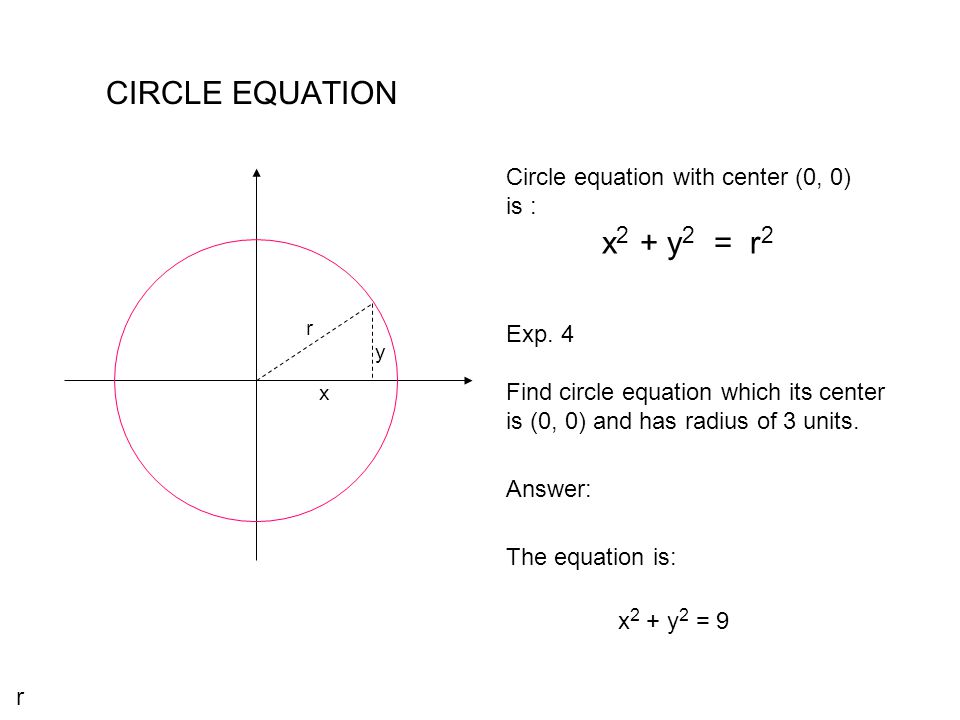
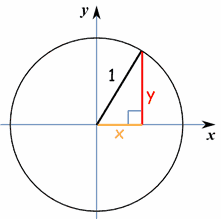
This means that, using Pythagoras' theorem, the equation of a circle with radius r and centre (0, 0) is given by the formula \(x^2 y^2 = r^2\) Example Find the equation of a circle withIt shows all the important information at a glance the center (a,b) and the radius rGiven that point (x, y) lies on a circle with radius r centered at the origin of the coordinate plane, it forms a right triangle with sides x and y, and hypotenuse r This allows us to use the Pythagorean Theorem to find that the equation for this circle in standard form is x 2 y 2 = r 2



Geometrical Definition Of A Circle A Circle Is



Equation Of Tangent To Circle X 2 Y 2 8x 10x 128 0 Ib Math Gcse Youtube
The equation of a circle can be given in a more general form, namely Ax2Ay2 BxCyD = 0 A x 2 A y 2 B x C y D = 0 If given such an equation, we can try to complete the square in theThis video explains how to write the general form of a circle in standard form and then graph the circlehttp//mathispower4ucom73 Equation of a tangent to a circle (EMCHW) On a suitable system of axes, draw the circle \(x^{2} y^{2} = \) with centre at \(O(0;0)\) Plot the point \(T(2;4)\)



Scarpelli Assignment 2




How To Graph A Circle 4 Easy Steps Equations Examples Video
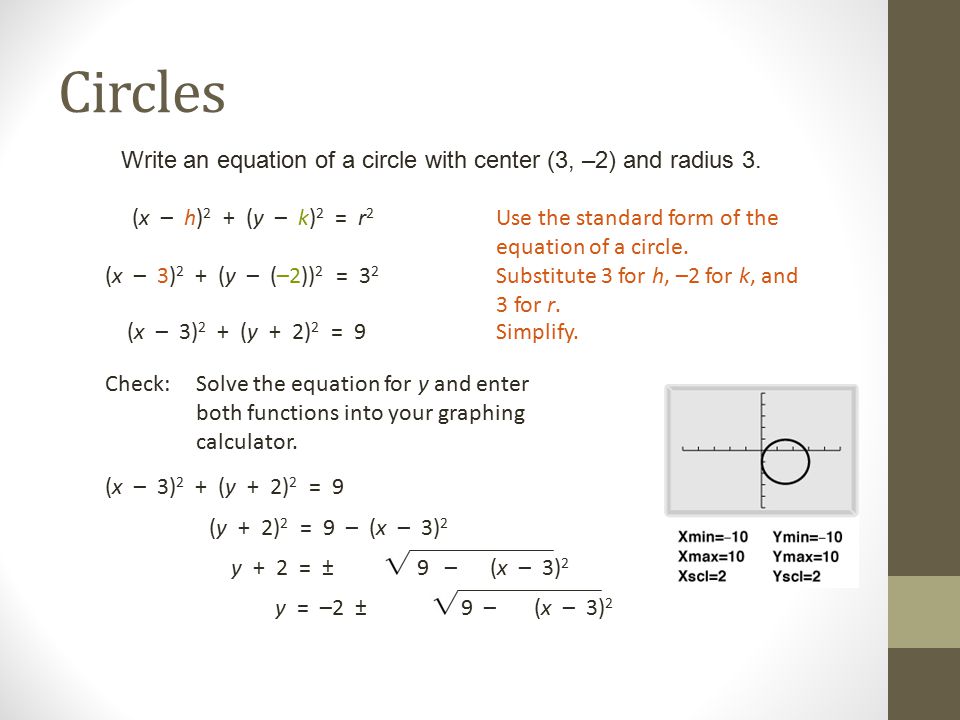
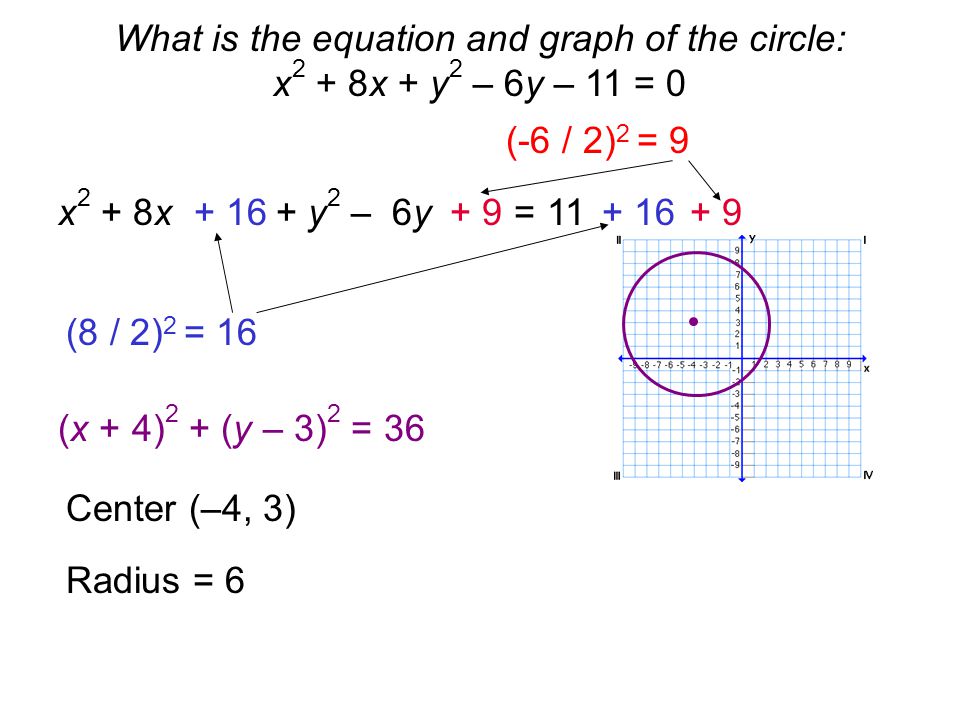
Equation of a Circle The technique of completing the square is used to turn a quadratic into the sum of a squared binomial and a number ( x – a) 2 b The centerradius form of the circle equation is in the format ( x – h) 2 ( y – k) 2 = r2, with the center being at the point ( h, k) and the radius being " r "By "touches" I take it the problem intends tangency, or "touches at exactly one point" If this is the case the answer can be gotten by the quadratic formula, or actually just its discriminant32 latexx^{2}y^{2}14x13=0/latex 33 Explain the relationship between the distance formula and the equation of a circle 34 In your own words, state the definition of a circle 35 In your own words, explain the steps you would take to change the general form of the equation of a circle to the standard form



Circle Equations Harder Example Video Khan Academy
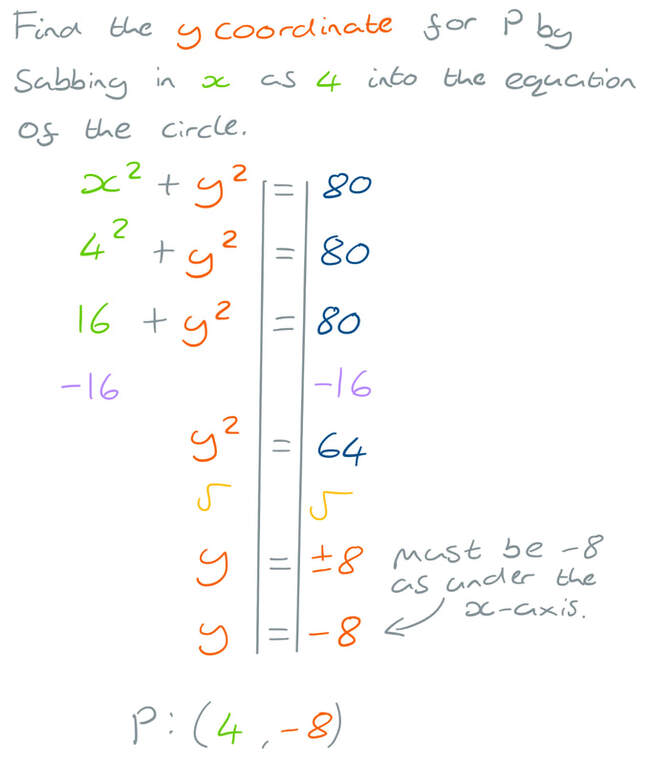



Q28 Answers Paper 3 June 18 Aqa Gcse Maths Higher Elevise
Given circle equation #x^2 y^2 4x 8y 13 = 0# The standard form of circle equation is #(xh)^2 (yk)^2 = r^2# where center # = (h, k)#, #" "r = # radius Use completing of the square to find the equation of the circle First combine the #x# terms and the #y# terms and put the constant(s) on the right side of the equationExplanation The general equation of a circle is (x – h) 2 (y – k) 2 = r 2, where (h, k) represents the location of the circle's center, and r represents the length of its radius Circle A first has the equation of (x – 4) 2 (y 3) 2 = 29 This means that its center must be Applying the definition of circle The definition of the circle is "all the point with a fixed distance from the center" You have to translate this in a formula The distance between two points in the plane with coordinates (x_1, y_1) and (x_2, y_2) is d=sqrt((x_1x_2)^2(y_1y_2)^2) Reading our definition of circle we want that one of the two points is always the center and the



1



1 Objectives 4 1 Circles A Determine The Equation Of A Circle B Determine The Centre And Radius Of A Circle By Completing The Square C Find The Ppt Download
A circle with center C has equation x^2 y^2 12x 6y = 0 a) By using the method of completing the square, find the coordinates of C and the radius of the circle b) The origin O is the midpoint of a chord PQ of this circle i) Show that the gradient of the chord PQ is 2It is the same idea as before, but we need to subtract a and b (x−a) 2 (y−b) 2 = r 2 And that is the "Standard Form" for the equation of a circle!X2 y2 − 4x − 6y − 12 = 0 x 2 y 2 4 x 6 y 12 = 0 This is the General Form of a circle You can recognize it because the two leading terms will always be x2 x 2 and y2 y 2 The generic General Form Equation looks like this x2 y2 Ax By C = 0 x 2 y 2 A x B y C = 0



How Do You Find The Center And Radius Of The Circle X 2 Y 2 2x 3 0 Socratic



Solved Graph Each Equation X 2 Y 2 100
Answer and Explanation 1 Given equation of a circle is x2y2 =121 (Eq1) (Eq1) x 2 y 2 = 121 We know that equation of a circle in the standard form is given as (x−h)2(y−k)2 = r2 (EqBasic Equation of a Circle (Center at 0,0) A circle can be defined as the locus of all points that satisfy the equation x 2 y 2 = r 2 where x,y are the coordinates of each point and r is the radius of the circle OptionsSubtract y^ {2} from both sides Subtract y 2 from both sides x^ {2}=r^ {2}y^ {2} x 2 = r 2 − y 2 Take the square root of both sides of the equation Take the square root of both sides of the equation x=\sqrt {\left (ry\right)\left (yr\right)} x=\sqrt {\left (ry\right)\left (yr\right)}



Circles Find The Missing Value To Complete The Square 6 X 2 2x 7 X 2 4x 8 X 2 6x Circles Warm Up Find The Missing Value To Complete Ppt Download



Equation Of A Circle Mathematics A Level Revision
Equation of the circle which passes through the centre of the circle x^2 y^2 8x 10y 7 = 0 and is concentric with the circle 2x^2 2y^2 8x 12y 9 = 10 isCircleradiuscalculator radius x^2y^2=1 en Related Symbolab blog posts Practice Makes Perfect Learning math takes practice, lots of practice Just like running, it takes practice and dedication If you wantFor example, suppose ( x 2 ) 2 ( y 3 ) 2 = 4 2 is an equation of a circle The center of this circle is located at ( 2 , 3 ) on the coordinate system and the radius is 4 How to derive the standard form of an equation of a circle Start with the circle you see below Then put the circle on the coordinate system



Recognise And Use X2 Y2 R2 Ppt Download




Circle Equation Diameter Circle
The standard equation of a circle is (xh) 2 (yk) 2 =r 2 Where (h, k) is the coordinates of the center, and r is the radius of the circle Remember that the value of r is always positive Let's see some examples based on the standard formAlgebra > Graphs> SOLUTION Write an equation that shifts the circle x^2y^2=16 to the left 2 units and downward 5 unit Sketch the graph and state the center and radius of the standard circle Sketch the graph and state the center and radius of the standard circleThe function $f(t) = \left(a\cos t, a\sin t\right)$ for $t \in 0,2\pi$ is a parametric description of the circle $x^2 y^2 = a^2$
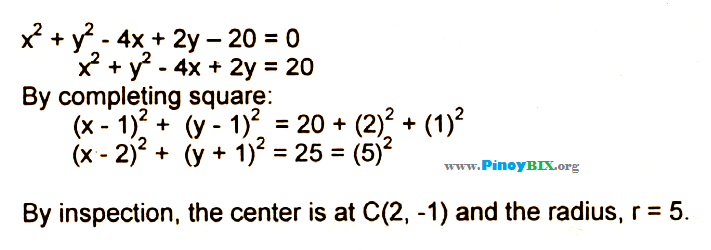



Solution The Equation X 2 Y 2 4x 2y 0 Describes
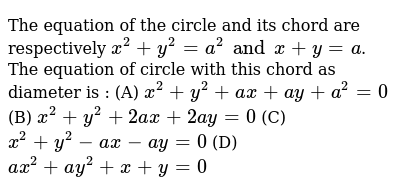



The Equation Of The Circle And Its Chord Are Respectively X 2 Y
X^2y^2=1 radius\x^26x8yy^2=0 center\(x2)^2(y3)^2=16 area\x^2(y3)^2=16 circumference\(x4)^2(y2)^2=25 circleequationcalculator x^2y^2=1 enExample 1 Find the focus and directrix and graph the parabola whose equation is y = 2x2 Solution Step 1 Analyze the problem Since the quadratic term involves x, the axis is vertical and the standard form x2 = 4py is used Step 2 Apply the formulaExample A circle passes through points A(2, 4) and B(2, 6) and its center lies on a line x 3y8 = 0 Find equation of the circle Solution The intersection of the chord AB bisector and the given line is the center S of the circle, since the bisector is normal through the midpoint M, then




C Is The Circle With The Equation X 2 Y 2 1 Brainly Com




Equation Of Circle 2 Youtube
For instance, to graph the circle x2 y2 = 16, follow these steps Realize that the circle is centered at the origin (no h and v) and place this point there Calculate the radius by solving for r Set r2 = 16 In this case, you get r = 4 Plot the radius points on the coordinate plane You count out 4 in every direction from the center (0, 0Derive the Area of a Circle Using Integration (x^2y^2=r^2) Watch later Share Copy link Info Shopping Tap to unmute If playback doesn't begin shortly, try restarting your device Up nextEQUATION OF A CIRCLE The equation of a circle comes in two forms 1) The standard form (x h) 2 (yk) 2 = r 2 2) The general form x 2 y 2 Dx Ey F = 0, where D, E, F are constants




Q28 Answers Paper 3 June 18 Aqa Gcse Maths Higher Elevise



How Do You Find The Center And Radius Of The Circle Given X 2 Y 2 144 Socratic
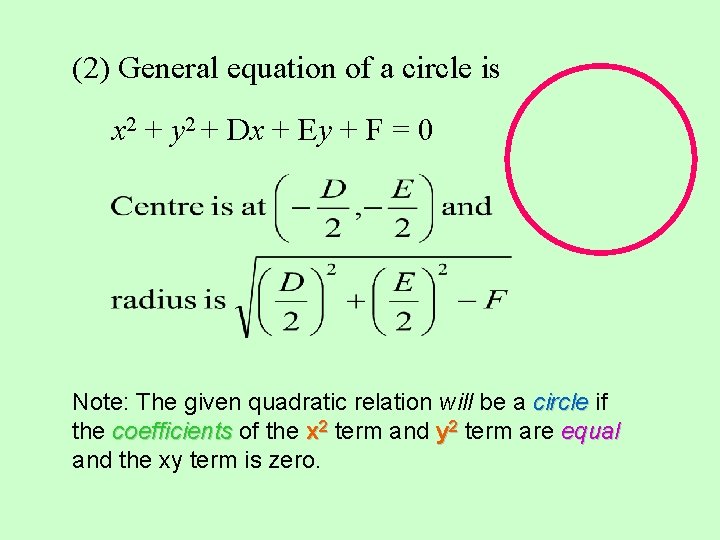
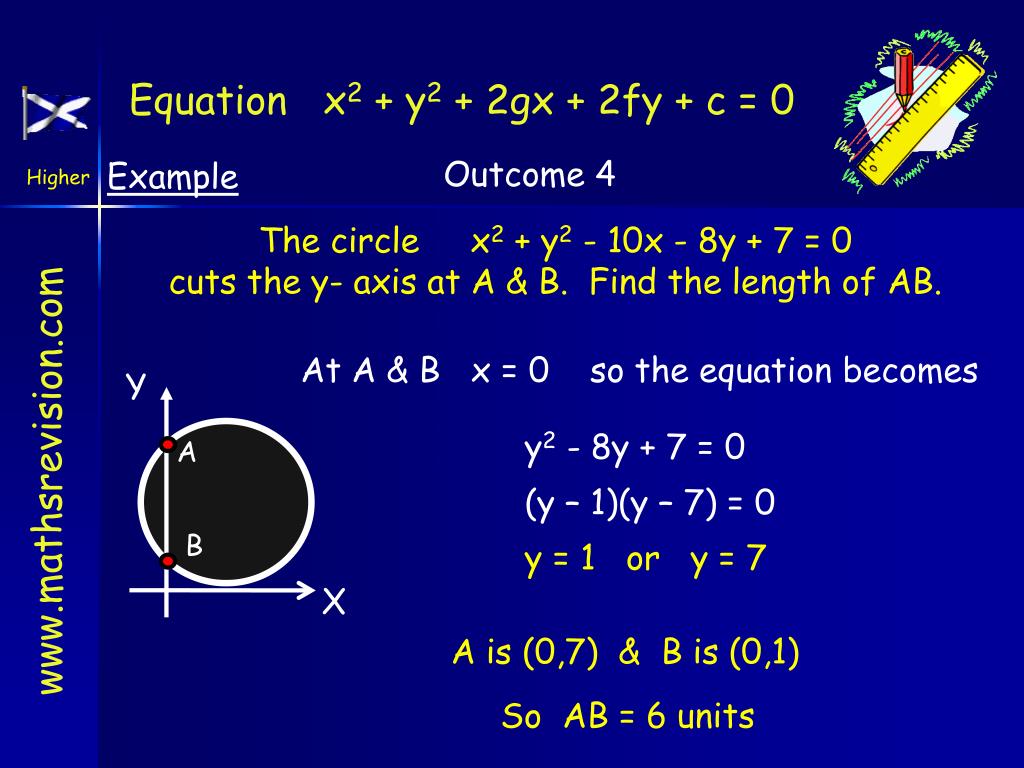
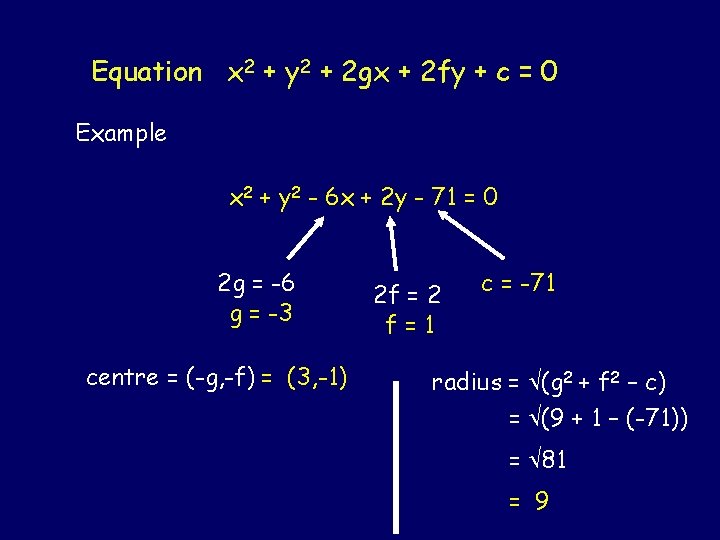
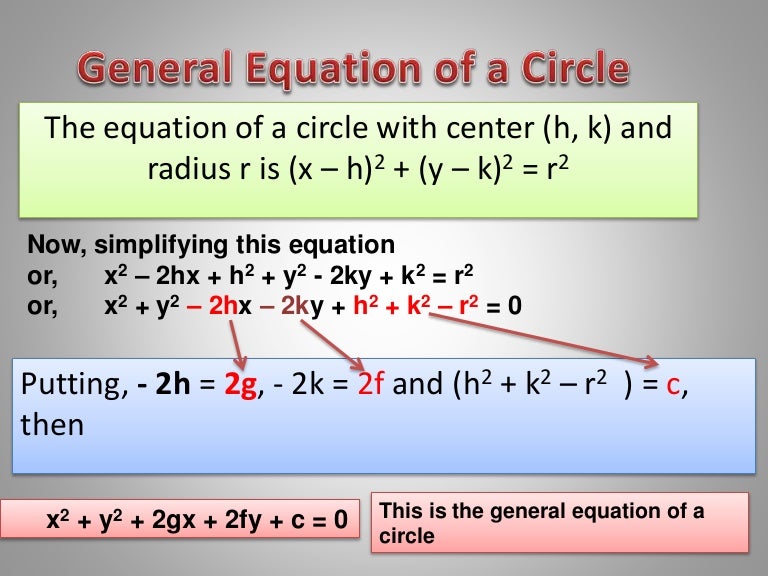
Find the Center and Radius x^2y^22x=0 Complete the square for Tap for more steps Use the form , Find the value of using the formula Tap for more steps Simplify each term Tap for more steps Raise to the power of Multiply by Match the values in this circle Equation of a Circle in General Form The general equation of any type of circle is represented by x2 y2 2gx 2fy c = 0, for all values of g, f and c Adding g2 f2 on both sides of the equation gives,The general equation of a circle is x2y2 2gx 2f yc= 0, where the centre is given by the radius byr=f2c The equation can(−g,−f)andpg2−be recognised because it is given by a quadratic expression inbothxandywith noxyterm,and where the coefficients of x2andy2are equal




Which Graph Shows The Graph Of A Circle With Equation X 2 Y 4 2 16 Brainly Com



Www Npsk12 Com Cms Lib Va Centricity Domain 37 Geometry Pdf
Find the Center and Radius x^2y^26x8y24=0 Subtract from both sides of the equation the form , to find the values of , , and Consider the vertex form of a parabola Substitute the values of and into the formula Simplify the right side Tap for more steps Cancel the common factor The variable represents the radius of the circleEquation of a Circle State the radius and center of the circle with equation 16 = (x – 2)2 (y – 3)2 The numerical side, the 16, is the square of the radius, so it actually indicates 16 = r2 = 4 2, so the radius is r = 4 Reading from the squaredvariable parts, the center is at (h, k) = (2, 3)X2 y2 = r2 x 2 y 2 = r 2 For example, a circle with a radius of 7 units and a center at (0, 0) (0, 0) looks like this as a formula and a graph x2 y2 = 49 x 2 y 2 = 49 How To Graph A Circle



Circles
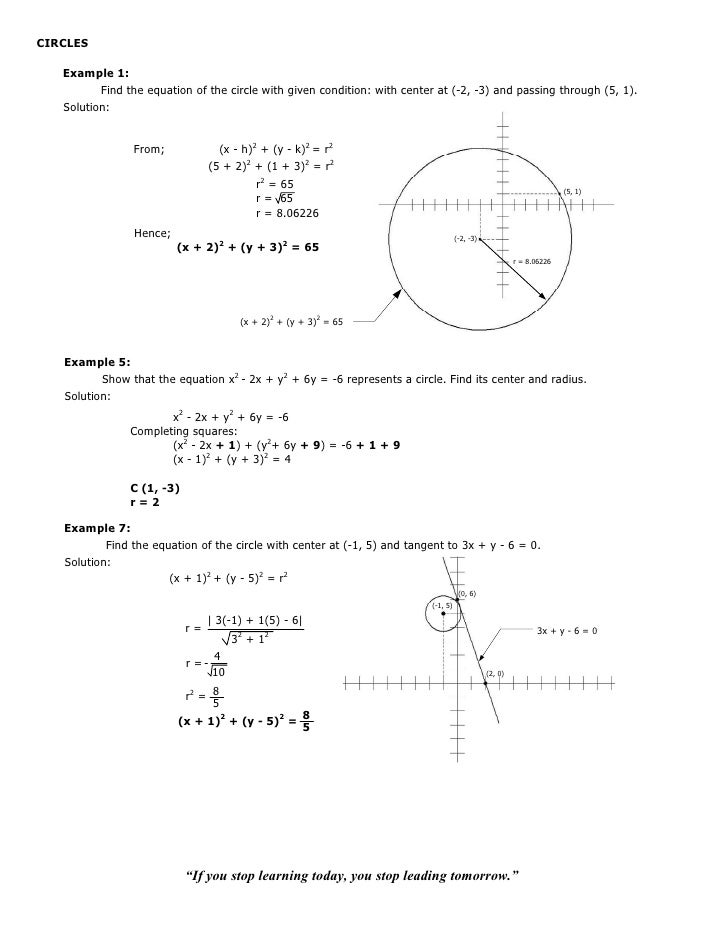



003 Circle
General Equation of a Circle The general equation of a circle is given by x 2 y 2 2gx 2fy c = 0, where centre of the circle = ( g, – f) Radius of the circle = √ g 2 f 2 – c If g 2 f 2 – c > 0, then the radius of the circle is real and hence the circle is also real;Find the circumference of the circle x 2 y 2 = 4 by using the formula for arc length of a curve Verify your answer by using the circumference formula for a circle Expert Answer Previous question Next question Get more help from Chegg Solve itThe center is at the origin The equation of a circle in standard form is (x−h)² (y−k)²=r² Where r is the radius and (h,k) is center If either h or k is missing, then its value must be 0 Thus, if both are missing the circle must be centered at the origin, (0,0) Comment on Just Keith's post "The center is




Find The Center And Radius Of Each Circle Whose Chegg Com




The Line L Is Tangent To The Circle With Equation X 2 Y 2 10 At The Point P Determine The Equation Brainly Com
An equation which can be written in the following form (with constants D, E, F) represents a circle x 2 y 2 Dx Ey F = 0 This is called the general form of the circle Example 4 Find the centre and radius of the circle x 2 y 2 8x 6y = 0 Sketch the circle




An Example Of A Hyperbola Of Equation X 2 A 2 Y 2 B 2 1 With Download Scientific Diagram



1



Find The Center Of The Circle Using Endpoints Of Diameter Geeksforgeeks




How To Graph A Circle 4 Easy Steps Equations Examples Video



Circles Intro
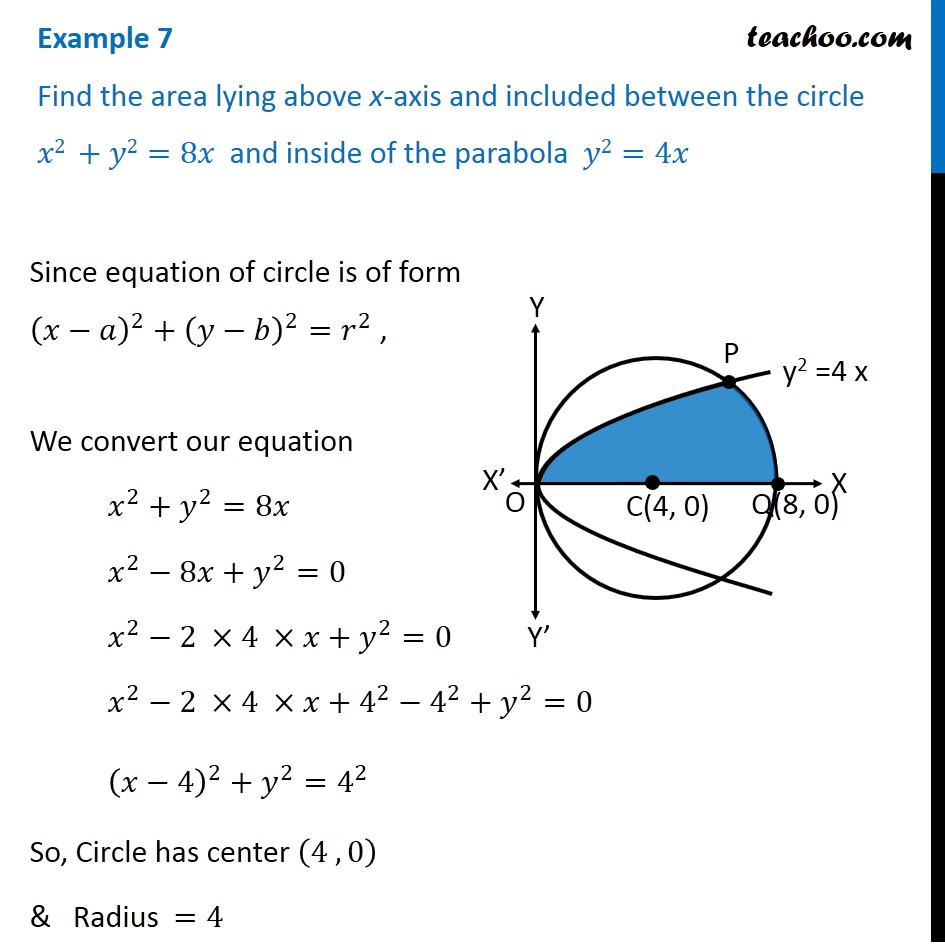



Example 7 Find Area Lying Above X Axis Included B W Circle
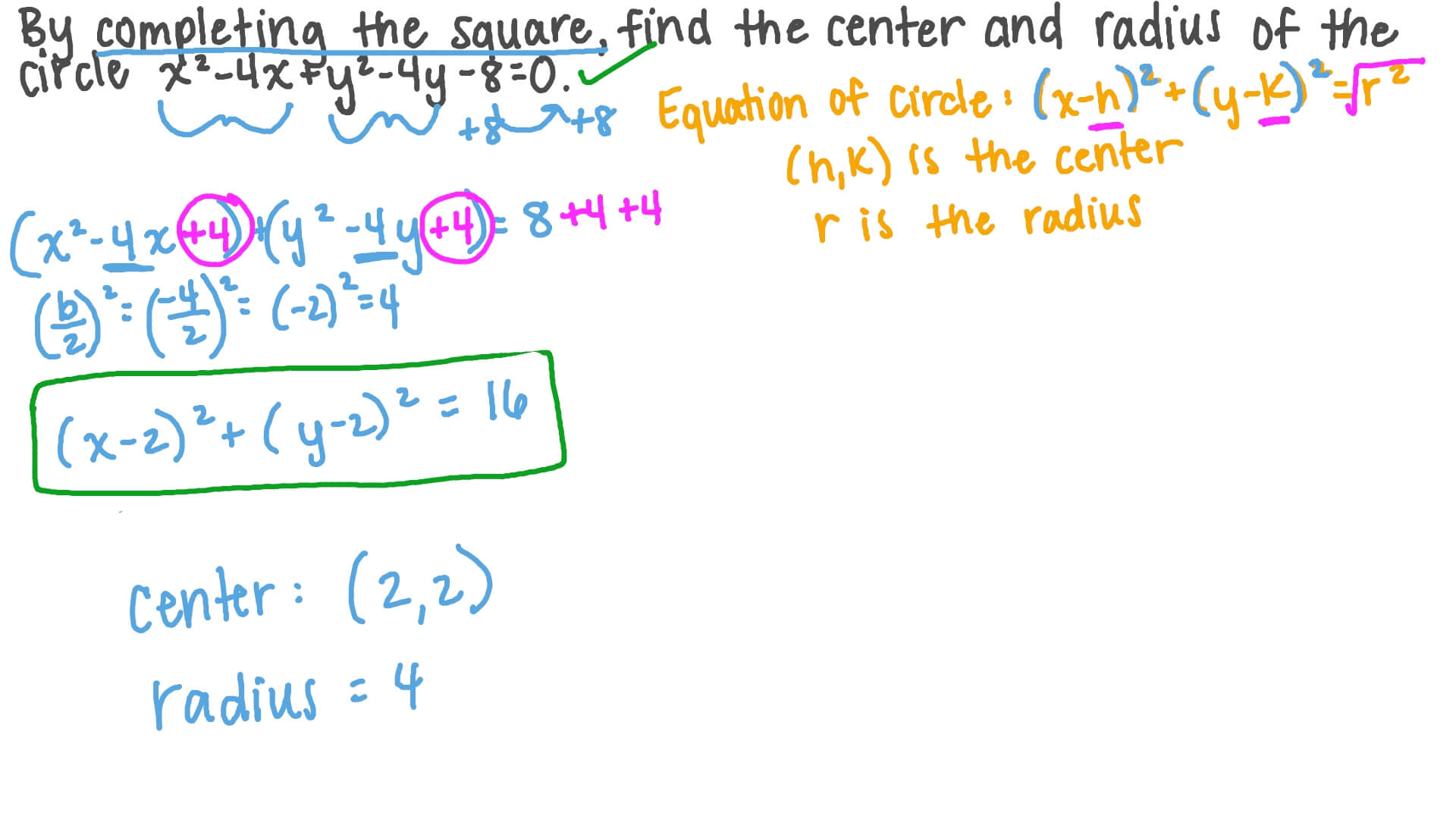



Question Video Finding The Center And Radius Of A Circle By Completing The Square Nagwa




Recognise And Use X2 Y2 R2 Ppt Download




Graph The Circle X 2 Y 2 8x 4y 11 0 Study Com



Learn Alternate Locus Circle Meaning Concepts Formulas Through Study Material Notes Embibe Com



Ppt Higher Unit 2 Powerpoint Presentation Free Download Id
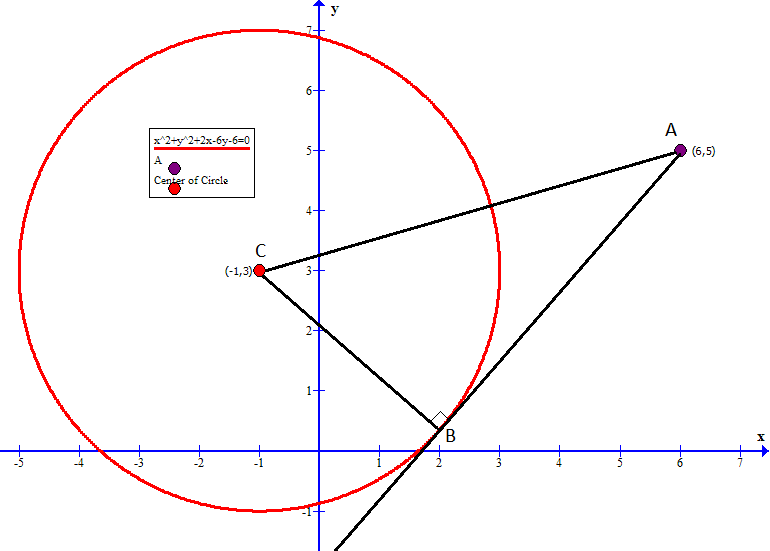



Given That A Circle Has An Equation Of X 2 Y 2 2x 6y 6 0 What Is The Exact Length Of Ab Given That A Tangent Is Drawn From A 6 5 And Touches The Circle At B Socratic




Show That The Equation X 2 Y 2 X Y 0 Represents A Circle Find Its Center And Radius



Solution Given The Equation Of A Circle X 2 Y 2 10x 4y 13 0 Find Its Center And Radius I Am Having Trouble Please Help



Circle Equations The Graphical Form Of The Circle




Q1 A Circle Has Equation X2 Y2 4x 14 0 A Chegg Com




Find The Parametric Equations Of The Circles X 2 Y 2 16




Circle Equations




Recognise And Use X2 Y2 R2 Ppt Download




Graph Of This Integral Is It A Half Circle Or Quarter Circle Mathematics Stack Exchange



Given The Equation X 2 Y 2 8x 2y 8 0 A Chegg Com



Example 1 Find Area Enclosed By Circle X2 Y2 Examples




Show That The Equation X 2 Y 2 X Y 0 Represents A Circle
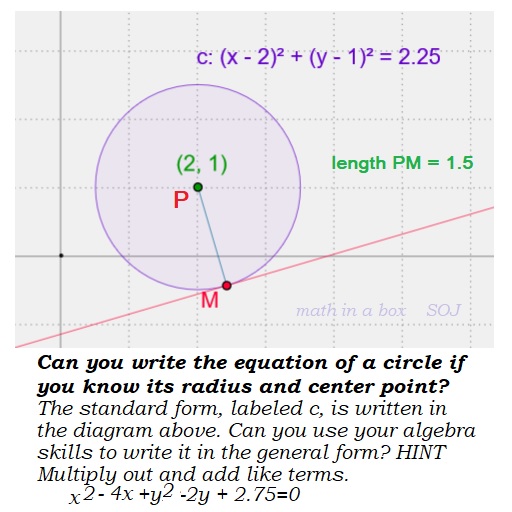



Circles In Algebra Math In A Box Lessons For Algebra Geometry And Precalculus




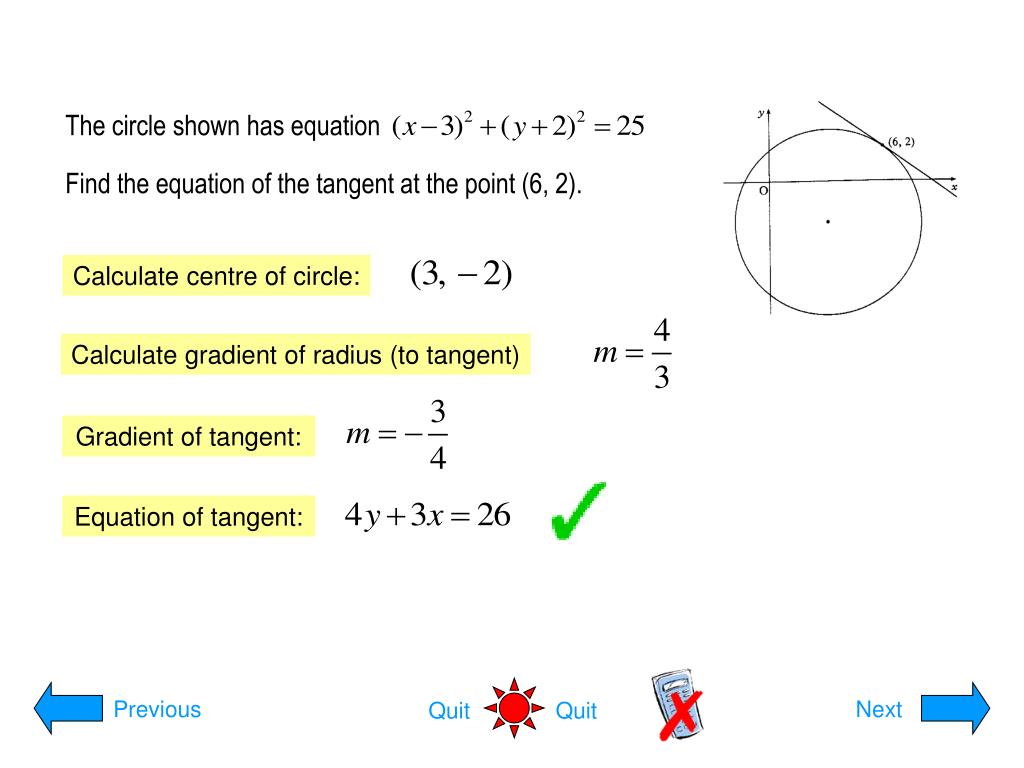
A Circle Has The Equation X 2 Y 2 25 Write An Equation Of The Line Tangent Youtube



Circle




Rd Sharma Solutions For Class 11 Maths Chapter 24 The Circle Download Free Pdf Available




Implicit Differentiation




Equation Of Circles A Plus Topper



Solution Find The Center And Radius Of The Circle X 2 Y 2 49 I 39 M Not Sure But I Have Used The Formula X H 2 Y K 2 Sq Root Of 49 And




The Circle With Equation X 2 Y 2 1 Intersects The Line Y 7x




Circle Equations
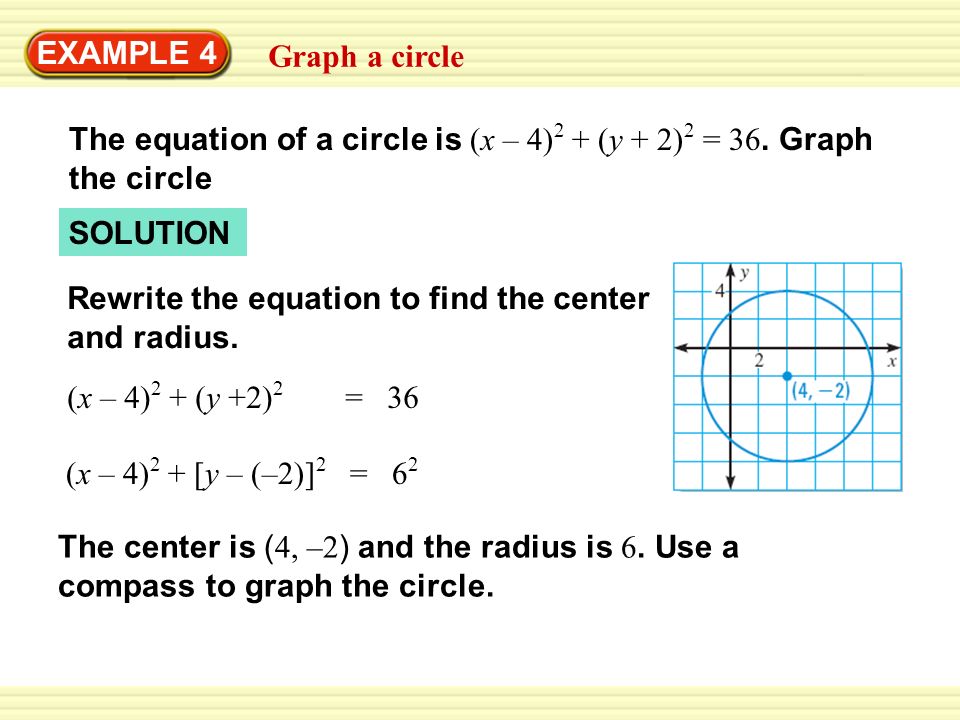



Example 4 Graph A Circle The Equation Of A Circle Is X 4 2 Y 2 2 36 Graph The Circle Solution Rewrite The Equation To Find The Center And Ppt Download



Circle Equations The Graphical Form Of The Circle
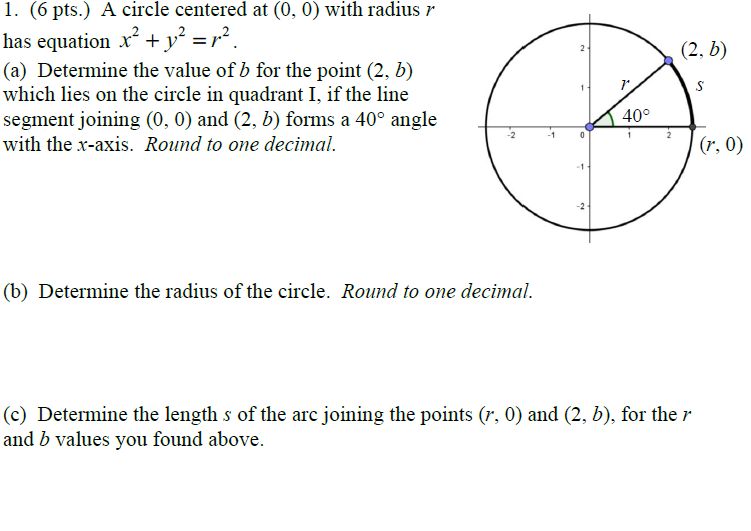



A Circle Centered At 0 0 With Radius R Has Chegg Com



In The Diagram Below M Is The Centre Of The Circle Having The Equation X 2 Y 2 6 X 2 Y 8 0 The Circle Passes Through R 0 4 And N P Q R Widehat M N 90 Circ
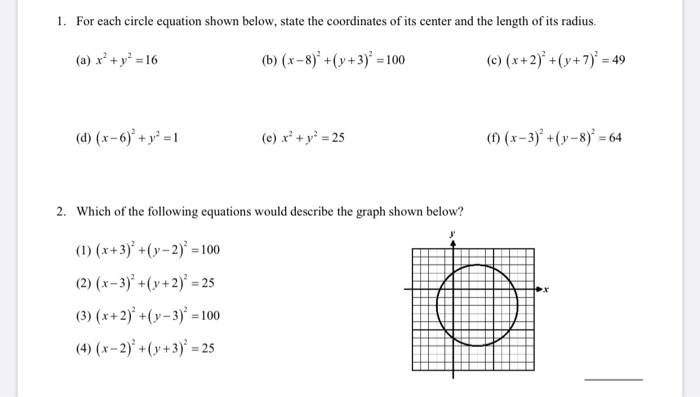



1 For Each Circle Equation Shown Below State The Chegg Com



1 X Y 49 Determine The Center And The Radius Of The Circle That Is Defined By Each Of The Following Equations Then Illustrate Each Circle On The Coordinate Plane How Quora



Solution Sketch The Circle Whose Equation Is X2 Y2 100 Using The Same System Of Coordinate Axes Graph The Line X 3y 10 Which Should Intersect The Circle Twice At A 10 0




Why Is Math X 2 Y 2 R 2 Math The Equation Of A Circle Quora
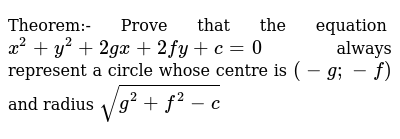



Theorem Prove That The Equation X 2 Y 2 2gx 2fy C 0 Always Rep




If One Of The Diameters Of The Circle Given By The Equation X



Www Npsk12 Com Cms Lib Va Centricity Domain 37 Geometry Pdf




Pin On Equations For Shapes
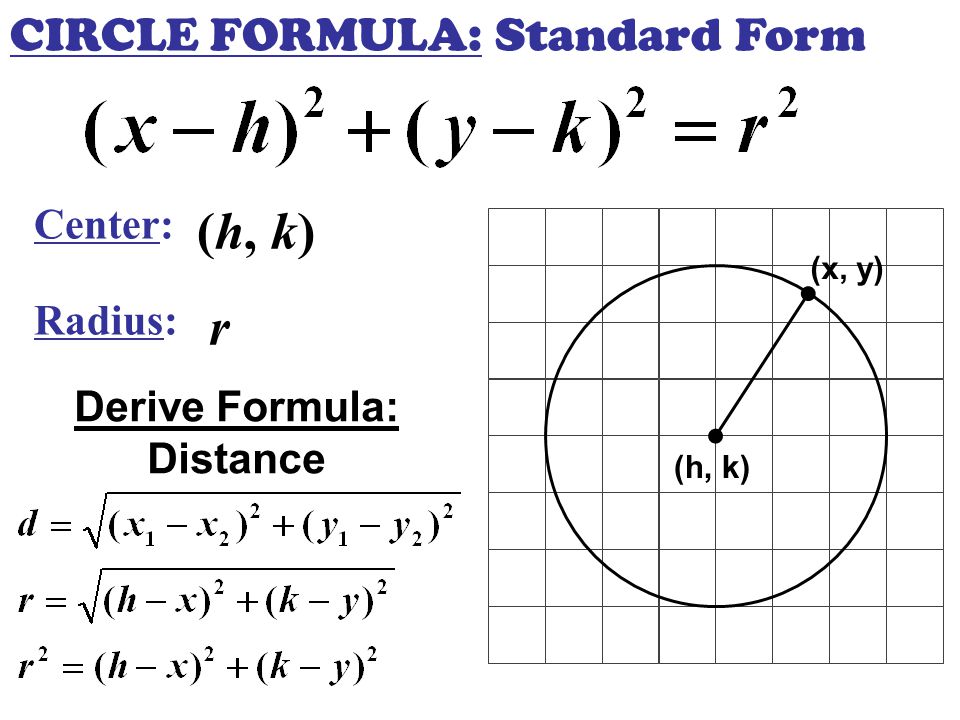



Midpoint Formula Distance Formula X 1 Y 1 X 2 Y 2 1 3 2 And 7 8 2 2 5 And 4 10 1 1 2 And 4 6 2 2 5 And 3 7 Coordinate Ppt Download
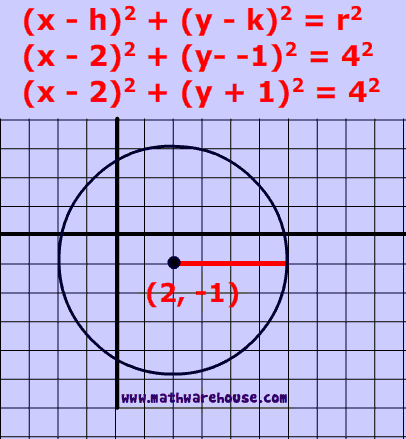



Equation Of A Circle In Standard Form Formula Practice Problems And Pictures How To Express A Circle With Given Radius In Standard Form




What Is C In Circle Equation
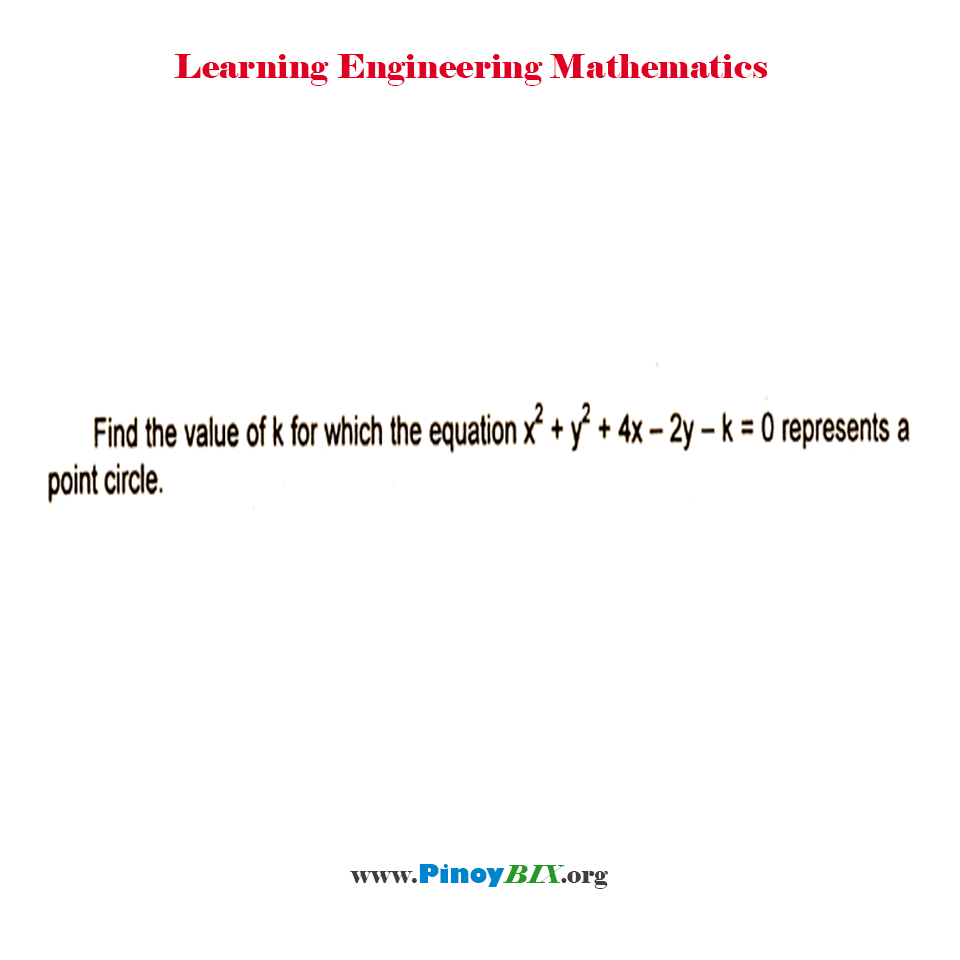



Solution Find The Value Of K For Which The Equation X 2 Y 2 4x 2y K 0



Show That The Equation X 2 Y 2 1 2 X 2y 1 16 Chegg Com
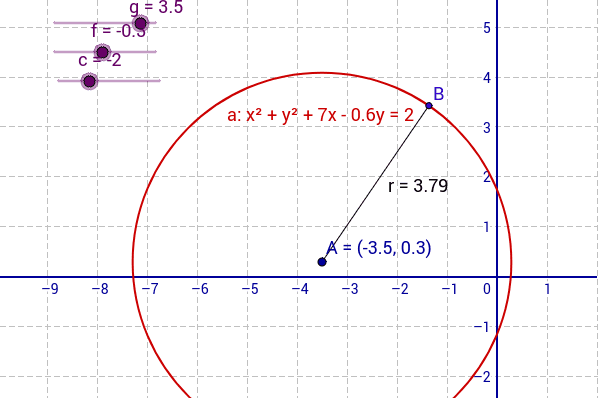



Equation Of Circle X 2 Y 2 2gx 2fy C 0 Geogebra



Circles Page 156 Lingkaran Halaman Ppt Download



Circle Equations The Graphical Form Of The Circle
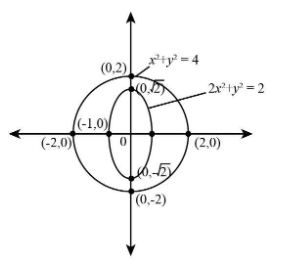



Find Common Tangent Of X2 Y2 4 And 2x2 Y2 2 Is Class 12 Maths Cbse



The Circle



C O N I C S E C T I O N S Part 2 The Circle Circle Ellipse X H 2 Y K 2 R 2 Ellipse X Ypoints On The Circle H Kthe Center Of The Circle Rthe Ppt Download



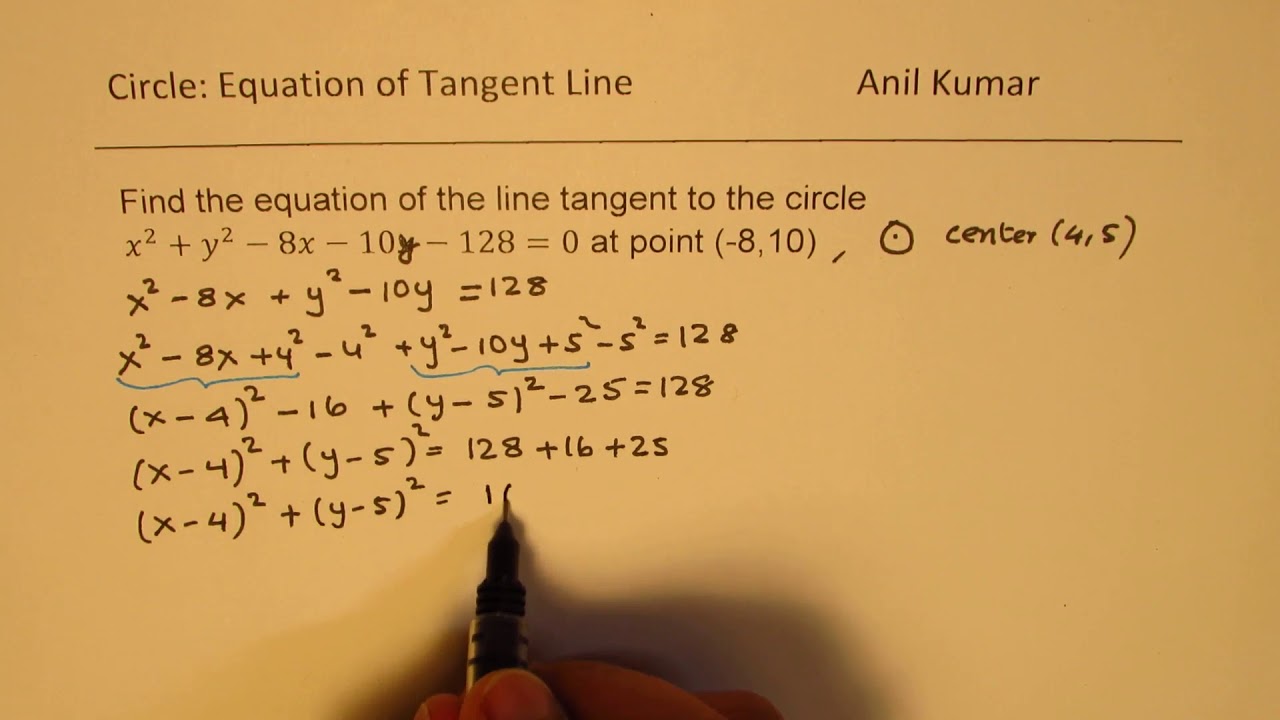
View Question Write The Equation Of The Line Tangent To The Circle X 2 Y 2 169 At The Point 5 12



If One Of The Diameters Of The Circle Given By The Equation X 2 Y 2 4x 6y 12 0 Is A Chord Of Sarthaks Econnect Largest Online Education Community




Describe All Integral Solutions Of The Equation X 2 Y 2 2z 2 Such That X Y Z 0 Gcd X Y Z 1 And X Y Mathematics Stack Exchange



Graphing This Circle Equation X 2 Y 2 14x 4y 0 Mathskey Com



Day 10 Equations Of Circles



Circle Equations The Graphical Form Of The Circle




Circle Equations



Circle Equations




Circles



Solution Find The Value Of K For Which The Equation X 2 Y



1



What Is The Area Of A Circle If The Circle Is Described Algebraically By The Equation X 2 Y 2 6x 8y 21 0 Quora




Circle Equations The Graphical Form Of The Circle



Graphing This Circle Equation X 2 Y 2 14x 4y 0 Mathskey Com



Ppt Circle Equations Powerpoint Presentation Free Download Id




Circle Has The Equation X 2 Y 2 2x 2y 14 0 How Do You Graph The Circle Using The Center H K Radius R Socratic




How To Construct The Graph Of X 2 Y 2 9 Quora




Walkthrough Of This Problem Gcse



1



Solution Find The Equation Of The Circle Concentric With The Circle X 2 Y 2 6x 2y 11 0 And Passing Through The Centre Of The Circle X 2 Y 2 16
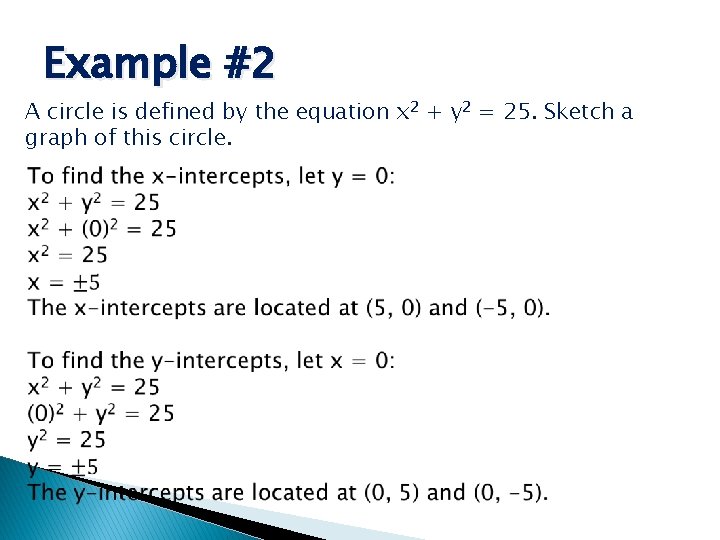



2 3 Equation Of A Circle How Is



How To Find The Center And Radius Of This Equation X2 Y2 6x 16 0 Quora



General Equation Of A Circle



0 件のコメント:
コメントを投稿